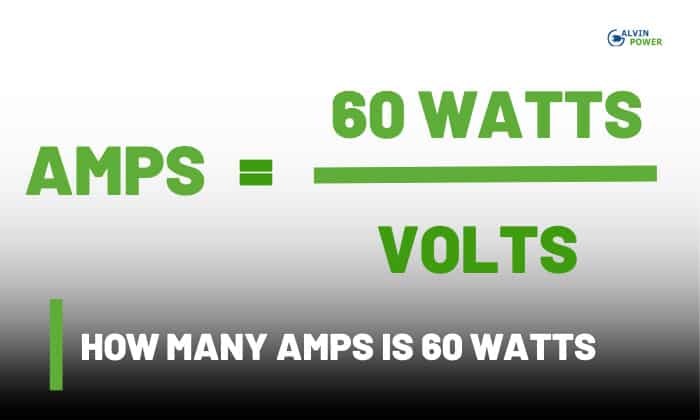
60 watt devices like incandescent bulbs must be accounted for if you plan to install them on a circuit. One thing is to check how many amps is 60 watts.
For DC circuits, 60 watts may be around 5 amps or 2.5 amps at 12 volts or 24 volts respectively. For single-phase AC, it may be at least 0.5 amps at 120 volts and at least 0.25 amps at 240 volts. For three-phase 208 volts AC, it’s at least 0.17 amps.
Table of Contents
60 Watts Convert to Amps at Different Voltages
Here is a chart that converts watts to amps. It lists 60 amps as well as other voltages. The list contains amps at different volts for both DC (direct current) connections and single-phase AC (alternating current) connections with three power factors:
| Power (watts) | DC | AC single phase | ||||||
| At 12V | At 24V | At 120V | At 240V | |||||
| – | – | PF = 1 | PF = 0.85 | PF = 0.5 | PF = 1 | PF = 0.85 | PF = 0.5 | |
| 35 | 2.92 | 1.46 | 0.29 | 0.34 | 0.58 | 0.15 | 0.17 | 0.29 |
| 40 | 3.33 | 1.67 | 0.33 | 0.39 | 0.67 | 0.17 | 0.20 | 0.33 |
| 45 | 3.75 | 1.88 | 0.38 | 0.44 | 0.75 | 0.19 | 0.22 | 0.38 |
| 50 | 4.17 | 2.08 | 0.42 | 0.49 | 0.83 | 0.21 | 0.25 | 0.42 |
| 55 | 4.58 | 2.29 | 0.46 | 0.54 | 0.92 | 0.23 | 0.27 | 0.46 |
| 60 | 5.00 | 2.50 | 0.50 | 0.59 | 1.00 | 0.25 | 0.29 | 0.50 |
| 65 | 5.42 | 2.71 | 0.54 | 0.64 | 1.08 | 0.27 | 0.32 | 0.54 |
| 70 | 5.83 | 2.92 | 0.58 | 0.69 | 1.17 | 0.29 | 0.34 | 0.58 |
| 75 | 6.25 | 3.13 | 0.63 | 0.74 | 1.25 | 0.31 | 0.37 | 0.63 |
| 80 | 6.67 | 3.33 | 0.67 | 0.78 | 1.33 | 0.33 | 0.39 | 0.67 |
| 85 | 7.08 | 3.54 | 0.71 | 0.83 | 1.42 | 0.35 | 0.42 | 0.71 |
Convert 60 Watts to Amps for Different Types of Current
Electric supplies in residential settings in the US typically are 120/240 volt single-phase current. In commercial settings, though, 120/208 volt three-phase is widely used. Here is a chart that lists watts to amps conversion for three-phase power at 60 watts and other ratings:
| Power (watts) | AC three phase | |||||
| At 120V (line-to-neutral) | At 208V ( line-to-line) | |||||
| PF = 1 | PF = 0.85 | PF = 0.5 | PF = 1 | PF = 0.85 | PF = 0.5 | |
| 35 | 0.29 | 0.34 | 0.58 | 0.10 | 0.11 | 0.19 |
| 40 | 0.33 | 0.39 | 0.67 | 0.11 | 0.13 | 0.22 |
| 45 | 0.38 | 0.44 | 0.75 | 0.12 | 0.15 | 0.25 |
| 50 | 0.42 | 0.49 | 0.83 | 0.14 | 0.16 | 0.28 |
| 55 | 0.46 | 0.54 | 0.92 | 0.15 | 0.18 | 0.31 |
| 60 | 0.50 | 0.59 | 1.00 | 0.17 | 0.20 | 0.33 |
| 65 | 0.54 | 0.64 | 1.08 | 0.18 | 0.21 | 0.36 |
| 70 | 0.58 | 0.69 | 1.17 | 0.19 | 0.23 | 0.39 |
| 75 | 0.63 | 0.74 | 1.25 | 0.21 | 0.24 | 0.42 |
| 80 | 0.67 | 0.78 | 1.33 | 0.22 | 0.26 | 0.44 |
| 85 | 0.71 | 0.83 | 1.42 | 0.35 | 0.42 | 0.71 |
The next section will discuss the equations used in the conversion tables in detail. An alternative way to convert is to use a watts to amps calculator.
How to Convert
1. DC supply
In a DC supply, the voltage is constant, and current flows in a single direction. For DC current like the one supplied by car batteries, electric power may be expressed with the following equation:
\begin{equation}
Power\ (W) = voltage\ (V) \times current\ (A)
\end{equation}
We can determine the current from this equation if we rearrange it like the one below:
\begin{equation}
Current\ (A) = \frac{Power\ (W)}{Voltage\ (V)}
\end{equation}
From here, we can see that the unit of current 1 amp = watts / volts.
For example, to solve for the current draw of a 40-watt light bulb in a car powered by a 12-volt battery, the bulb will draw an estimated current of:
\begin{equation}
Current\ (A) = \frac{Power\ (W)}{Voltage\ (V)} = \frac{40\ W}{12\ V} = 3.33\ A
\end{equation}
Alternatively, this can be solved using an online amperage calculator. Just set it to “calculator DC” option.
2. AC single–phase supply
While a DC supply has constant voltage, an AC supply cycles and changes direction depending on the frequency (in the US, it’s 60 Hz). In the US, single-phase supply is 120/240 volts with 2 hot legs, 1 neutral and ground.
In some cases, however, the voltage cycle and the current cycle might not be in sync. This causes losses in useful power that the devices can use.
For AC supplies, the power factor should be considered. It is the ratio between the device’s power output (actual power) and its input (apparent power). It ranges from 0 (very poor) to 1 (ideal), and its formula is:
\begin{equation}
Power\ Factor = \frac{Actual\ power\ (W)}{Apparent\ power\ (VA)} = \frac{100\ W}{120\ VA} = 0.83
\end{equation}
Power factors can show how much of the input is used to generate the output. A perfect power rating of 1 means all of the input power is used for the output, and this is true for resistive loads like our 40-watt incandescent bulb, while a lower rating means that some energy is wasted. A good power factor is around 0.95 and above.
The actual current the equipment may draw from the outlet can be solved if we break down apparent power and rearrange the expression above to:
\begin{equation}
Apparent\ power\ (VA) = \frac{Actual\ power\ (W)}{Power\ Factor}
\end{equation}
\begin{equation}
Voltage\ (V) \times Current\ (A) = \frac{Actual\ power\ (W)}{Power\ Factor}
\end{equation}
\begin{equation}
Current\ (A) = \frac{Actual\ power\ (W)}{Power\ Factor \times Voltage\ (V)}
\end{equation}
For example, if we want to know the current drawn by a 400-watt fridge with an 0.85 power factor running on 110V AC, then:
\begin{equation}
Current\ (A) = \frac{Actual\ power\ (W)}{Power\ Factor \times Voltage\ (V)} = \frac{400\ W}{0.85\times 110\ V} = 4.28\ A
\end{equation}
Just like a DC supply, AC supplies can also be calculated. Just set the online calculator to “calculator AC”.
3. Three-phase supply
Unlike single-phase supplies, a three-phase supply has 3 hot legs instead of 2. Each leg carries a current that is 120 degrees out of phase from each other. The common configuration is 120/208V.
Each leg in the three-phase supply can carry 120 volts. If we connect a load to one leg of this supply, the load will encounter only one of the three currents with a line-to-neutral voltage (VL-N) of 120 volts. The line-to-neutral current for this setup may be calculated using the formula:
\begin{equation}
Current\ (A) = \frac{Actual\ power\ (W)}{3 \times Power\ Factor \times Voltage_{L-N}\ (V)}
\end{equation}
However, if we connect the load to two legs of this supply, the load will encounter a line-to-line voltage (VL-L) of 208 volts. The line-to-line current drawn will be:
\begin{equation}
Current\ (A) = \frac{Actual\ power\ (W)}{\sqrt{3} \times Power\ Factor \times Voltage_{L-L}\ (V)}
\end{equation}
For example, if we want to connect the 60-watt bulb with a power factor of 1 to the 208 volt connection, then the three phase watts to amps will be:\begin{equation}
Current\ (A) = \frac{Actual\ power\ (W)}{\sqrt{3} \times Power\ Factor \times Voltage_{L-L}\ (V)} = \frac{60}{\sqrt{3} \times 1 \times 208} = 0.17\ A
\end{equation}
Conclusion
How many amps is 60 watts? It depends on whether you connect it to a DC supply, single AC supply or three-phase AC supply. The current can range from 0.17 amps to 5 amps, depending on how you connect the load.
Of course, all of this planning will ensure that your 60-watt light bulb will not cause overloading in your circuit. Even though the load is small, safety shall always be the priority, and knowing the current draw is the first step to safe electrical use.
Related:

I am Edwin Jones, in charge of designing content for Galvinpower. I aspire to use my experiences in marketing to create reliable and necessary information to help our readers. It has been fun to work with Andrew and apply his incredible knowledge to our content.